- Joined
- Aug 18, 2008
- Messages
- 4,076
This is the first of several articles where I will attempt to sum up the basics of car handling. Pretty much every aspect can be expanded on, however in the interests of keeping it simple I'll only include the essential parts needed to understand the whole process and not concern you all with the full array of nuances and equations used to calculate everything. I have written this pretty much straight from memory so apologies if some of the physics terms are incorrect or the progression is a bit messy.
We'll start at the beginning with tyres. Tyres are the single most important aspect of the vehicles suspension system. They are the only point of contact between your car and the road, without them you cannot transfer force to the road to accelerate, brake or turn. For now though, we are only concerned with turning.
Tyres loosely adhere to the laws of dry sliding friction, which dictate that lateral force (Fl) is equal or less than the vertical load (Fv) multiplied by the co-efficient of friction (μ), a constant for the pair of materials in contact. (Note how theoretical grip is independent of surface area and so contact patch of the tyre.)
We can see that lateral force increases proportionally with increased vertical load, given a constant friction coefficient. However, rubber is a soft, deformable material, and as such it’s co-efficient of friction is not constant. What in fact happens is that as vertical load increases, the μ drops. The end result of this is that as vertical load increases, lateral force increases but at a decreasing rate. So if 100lbs vertical produces 100lbs lateral, 200lbs vertical only yields 150lbs lateral, 300lbs vertical yields 175lbs lateral and so on.
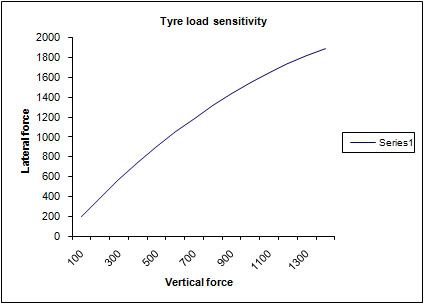
This relationship between vertical and lateral force is called load sensitivity. Load sensitivity varies greatly between different tyres, some may not be very sensitive over wide range of loads and temperatures, others may be more sensitive over certain temperature/load margins etc etc. The general trend is summarised by the following graph, we can see that grip tails off as load increases, eventually to a point where further increases actually reduce grip.
Lets now look at this with relation to a pair of tyres on an axle with 400lbs static vertical load, so 200lbs each. Going on my basic example above, our maximum potential lateral force in this condition is 150 + 150 = 300lbs potential grip.
Now lets transfer 100lbs from one side to the other, simulating a car in a corner, so we have one wheel on 300lbs vertical and one on 100lbs.
We get 175 + 100 = 275lbs potential grip from the tyre pair.
We can see from this that a pair of tyres makes the most grip when equally loaded, as the unloaded tyre will always lose grip faster than the loaded wheel gains it.
LOAD TRANSFER
With tyre basics covered, we now move onto the next aspect of handling, load transfer. Load transfer is the shift in vertical tyre load from the inside wheels to the outside wheels as a vehicle experiences lateral (sideways) acceleration in a turn. It also happens under longitudinal (forwards/backwards) acceleration but that is not of a concern in this discussion.
Load transfer is a result of a few basic variables in the vehicles design: track (tr),
height of the centre of gravity (cgh),vehicle mass (m) and acceleration (a). These four variables alone dictate how much load transfer a vehicle experiences, and are difficult to change aside from the weight. The formula for load transfer is:
So for a an EK9 experiencing 1G lateral acceleration, we get:
So that’s 827lbs or 376kg transferred from the inside wheels to the outside in a 1G corner.
What is important at this point is to differentiate between load transfer and roll. Load transfer is engineered into the vehicle, it cannot be drastically changed other than by changing the accelerative force acting upon the car. Since we are interested in going faster, what we actually want is more load transfer. Roll, as we will get onto later, is not so good. It is a product of load transfer, but it is easily changed by altering spring rates and anti-roll bars.
So, in our 1G corner, we have transferred 827lbs off of the inside wheels and loaded the outside wheels with it.
If we assume our EK9 to have 62% of its total mass over the front wheels, the static tyre loads would look like this:
With 1G lateral acceleration acting upon the car, and assuming the total load transfer to be distributed evenly between the two axles, they would look more like this:
As stated earlier, total load transfer is engineered into the vehicle and cannot be changed, however the F/R distribution of this load transfer can be altered, and is what we change when we alter spring rates and anti-roll bars. As you make one end proportionately stiffer than the other, you increase the amount of the total weight transfer that axle sees, and reduce the transfer the other sees.
This is where the relationship with the tyres comes in to play to form the 'balance' of the car with regards to over/understeer. You will recall how a pair of tyres makes most grip when equally loaded, and how the more load is transferred the less grip they can produce in total, well now we can work out the balance between front and rear load transfer to see which end is delivering the most grip and thus pushing towards understeer or oversteer.
At this point, I'll introduce the idea of 'front diagonal', a way of expressing the balance in load transfer between front and rear. Front diagonal is calculated as the load on the front outside tyre plus load on the inside rear, divided by the total vehicle mass.
FDiag = (fr + rl)/m
In this case, (1156.9 + 42.12) / 2398 = .50 = 50%
Since we split the 827lbs load transfer equally between front and rear axles, this is reflected in the 50% front diagonal. If we remember that a tyre pair loses grip with load transfer, this would translate into a neutral balance of front and rear grip as both axles will have lost grip at the same rate.
Let's say the front axle has stiffer springs, and so takes more load transfer, say 65% of total.
827lbs . 65% = 537.55lbs transferred on the front axle
827lbs . 35% = 289.45lbs transferred on the rear axle
Those tyre loads are now:
The front diagonal is now (1280.93 + 166.17) / 2398 = .603 = 60.3%
In this case, there is a bigger load differential at the front than there is the back, and since a bigger load differential means less grip, we can see that this car will understeer with the front making less grip than the rear.
There we have the basics making up vehicle balance, grip and understeer / oversteer.
I am happy to answer any questions, expand on any areas (if I can), and take constructive critisism on any aspects I may have got incorrect etc, otherwise, hope this has been of help to you.
In the next thread we’ll look at spring and damper selection.
Any reps greatly appreciated!
We'll start at the beginning with tyres. Tyres are the single most important aspect of the vehicles suspension system. They are the only point of contact between your car and the road, without them you cannot transfer force to the road to accelerate, brake or turn. For now though, we are only concerned with turning.
Tyres loosely adhere to the laws of dry sliding friction, which dictate that lateral force (Fl) is equal or less than the vertical load (Fv) multiplied by the co-efficient of friction (μ), a constant for the pair of materials in contact. (Note how theoretical grip is independent of surface area and so contact patch of the tyre.)
So the basic formula for dry sliding friction is Fl ≤ Fv . μ
We can see that lateral force increases proportionally with increased vertical load, given a constant friction coefficient. However, rubber is a soft, deformable material, and as such it’s co-efficient of friction is not constant. What in fact happens is that as vertical load increases, the μ drops. The end result of this is that as vertical load increases, lateral force increases but at a decreasing rate. So if 100lbs vertical produces 100lbs lateral, 200lbs vertical only yields 150lbs lateral, 300lbs vertical yields 175lbs lateral and so on.
This relationship between vertical and lateral force is called load sensitivity. Load sensitivity varies greatly between different tyres, some may not be very sensitive over wide range of loads and temperatures, others may be more sensitive over certain temperature/load margins etc etc. The general trend is summarised by the following graph, we can see that grip tails off as load increases, eventually to a point where further increases actually reduce grip.

Lets now look at this with relation to a pair of tyres on an axle with 400lbs static vertical load, so 200lbs each. Going on my basic example above, our maximum potential lateral force in this condition is 150 + 150 = 300lbs potential grip.
Now lets transfer 100lbs from one side to the other, simulating a car in a corner, so we have one wheel on 300lbs vertical and one on 100lbs.
We get 175 + 100 = 275lbs potential grip from the tyre pair.
We can see from this that a pair of tyres makes the most grip when equally loaded, as the unloaded tyre will always lose grip faster than the loaded wheel gains it.
LOAD TRANSFER
With tyre basics covered, we now move onto the next aspect of handling, load transfer. Load transfer is the shift in vertical tyre load from the inside wheels to the outside wheels as a vehicle experiences lateral (sideways) acceleration in a turn. It also happens under longitudinal (forwards/backwards) acceleration but that is not of a concern in this discussion.
Load transfer is a result of a few basic variables in the vehicles design: track (tr),
height of the centre of gravity (cgh),vehicle mass (m) and acceleration (a). These four variables alone dictate how much load transfer a vehicle experiences, and are difficult to change aside from the weight. The formula for load transfer is:
Lt = a . (cgh / tr) . m
So for a an EK9 experiencing 1G lateral acceleration, we get:
Lt = 1 . (20” / 58” . 2398lbs
. 2398lbs
Lt = 826.89lbs
Lt = 826.89lbs
So that’s 827lbs or 376kg transferred from the inside wheels to the outside in a 1G corner.
What is important at this point is to differentiate between load transfer and roll. Load transfer is engineered into the vehicle, it cannot be drastically changed other than by changing the accelerative force acting upon the car. Since we are interested in going faster, what we actually want is more load transfer. Roll, as we will get onto later, is not so good. It is a product of load transfer, but it is easily changed by altering spring rates and anti-roll bars.
So, in our 1G corner, we have transferred 827lbs off of the inside wheels and loaded the outside wheels with it.
If we assume our EK9 to have 62% of its total mass over the front wheels, the static tyre loads would look like this:
FR_______FL
743.4_____743.38
RR_______RL
455.6 _____455.62
743.4_____743.38
RR_______RL
455.6 _____455.62
With 1G lateral acceleration acting upon the car, and assuming the total load transfer to be distributed evenly between the two axles, they would look more like this:
FR_______FL
1156.9_____329.88
RR_______RL
869.12_____42.12
1156.9_____329.88
RR_______RL
869.12_____42.12
As stated earlier, total load transfer is engineered into the vehicle and cannot be changed, however the F/R distribution of this load transfer can be altered, and is what we change when we alter spring rates and anti-roll bars. As you make one end proportionately stiffer than the other, you increase the amount of the total weight transfer that axle sees, and reduce the transfer the other sees.
This is where the relationship with the tyres comes in to play to form the 'balance' of the car with regards to over/understeer. You will recall how a pair of tyres makes most grip when equally loaded, and how the more load is transferred the less grip they can produce in total, well now we can work out the balance between front and rear load transfer to see which end is delivering the most grip and thus pushing towards understeer or oversteer.
At this point, I'll introduce the idea of 'front diagonal', a way of expressing the balance in load transfer between front and rear. Front diagonal is calculated as the load on the front outside tyre plus load on the inside rear, divided by the total vehicle mass.
FDiag = (fr + rl)/m
In this case, (1156.9 + 42.12) / 2398 = .50 = 50%
Since we split the 827lbs load transfer equally between front and rear axles, this is reflected in the 50% front diagonal. If we remember that a tyre pair loses grip with load transfer, this would translate into a neutral balance of front and rear grip as both axles will have lost grip at the same rate.
Let's say the front axle has stiffer springs, and so takes more load transfer, say 65% of total.
827lbs . 65% = 537.55lbs transferred on the front axle
827lbs . 35% = 289.45lbs transferred on the rear axle
Those tyre loads are now:
FR_______FL
1280.93_____205.83
RR_______RL
745.07_____166.17
1280.93_____205.83
RR_______RL
745.07_____166.17
The front diagonal is now (1280.93 + 166.17) / 2398 = .603 = 60.3%
In this case, there is a bigger load differential at the front than there is the back, and since a bigger load differential means less grip, we can see that this car will understeer with the front making less grip than the rear.
There we have the basics making up vehicle balance, grip and understeer / oversteer.
I am happy to answer any questions, expand on any areas (if I can), and take constructive critisism on any aspects I may have got incorrect etc, otherwise, hope this has been of help to you.

In the next thread we’ll look at spring and damper selection.
Any reps greatly appreciated!
Last edited:
